(35a) Planetary Gravity-Assist and the Pelton Turbine

The most significant words of discovery are not "Eureka, I found it" but "Hmmm. That is funny."                Anonymous

Lester Pelton

Lester Pelton, the inventor of the device, was born in 1829 in Vermillion, Ohio, on the shores of Lake Erie. In 1850 he arrived in California with the gold rush migrants and became a fisherman on the Sacramento river. Around 1853 he gave up on fishing and started working in the gold mines of Camptonville, Nevada City and Grass Valley, on the Yuba river in the "mother lode country." In 1864 he became a carpenter and millwright.

By that time the search for gold had progressed from sifting creek gravel ("placer mining") to processing large amounts of ore--from gravel deposits on the surface and from hard-rock mining. This required power--to drive stamp mills that crushed the ore, to pump air into mine tunnels and later to provide electricity and compressed air. Some mines used steam engines, but these needed a large and constant supply of firewood.

Mountain creeks could also supply energy, but the amount of their water was rather limited, although it fell from great height. Therefore, instead of the slow (and inefficient) water wheels traditionally used by flour mills, which needed only a moderate height but used a large amount of water, mine owners installed turbines--wheels with cups around their circumference, on which a fast jet of water was directed, coming from a high-up reservoir.

According to a 1939 article by W. F. Durand of Stanford University in Mechanical Engineering, Pelton´s invention started from an accidental observation, some time in the 1870s. Pelton was watching a spinning water turbine when the key holding its wheel onto its shaft slipped, causing it to become misaligned. Instead of the jet hitting the cups in their middle, the slippage made it hit near the edge (drawing); rather than the water flow being stopped, it was now deflected into a half-circle, coming out again with reversed direction. Surprisingly, the turbine now moved faster

    Planetary gravity-assist,
    once more

v_final = –u + 2v = +20 + 40 = 60 mph

v_final = –u + 2v

1. The ball moves left-to-right and the paddle moves in the opposite direction. Then (–u) and v are both positive, so the magnitude of vfinal is always larger than the initial velocity (–u). The final energy
   E_final = (m/2) (v_final)² is increased.
   
   
2. The paddle does not move at all. Then v=0 and
   v_final = –u The final velocity has the same magnitude as the initial one, only the direction is reversed. The energy is unchanged.
   
   
3. Both ball and paddle move in the same direction, left-to-right, and the ball overtakes the paddle, then both u and v are negative. The final velocity
   v_final = –u + 2v is the sum of a positive number (-u) and a negative one (2v). Its magnitude is therefore less than the magniitude (-u) of the initial velocity: the ball comes out moving more slowly than before. Its energy is reduced.
   
   
4. In particular, if v = u/2, a negative number (e.g. v = –10 mph in the example given), then
   v_final = 0 and the ball just dribbles out with no velocity of its own.
   

Sometimes losing energy is just what is needed. Suppose a scientific probe is to be sent to the Sun. A spacecraft which has escaped the Earth´s gravity still circles the Sun at about 30 km/sec, and to escape the solar system it needs an added push of about 12 km/s. To reach the Sun is much harder: the spacecraft needs (at the very least) to lose all its orbital velocity, which requires a boost of 30 km/sec against the direction it is moving. Once all its velocity is lost, it falls freely sunward.

NASA´s Solar Probe, meant to approach the Sun within 4 solar radii (about 2% of the Sun-Earth distance), has similar requirements. As far as rocket power is concerned, the most economical way of achieving that mission (though not the fastest) may well be sending the spacecraft outwards towards Jupiter, 5 times more distant from the Sun. It would then make a tight loop around the planet, overtaking it in such a way that practically all its orbital velocity around the Sun is lost, and then fall towards the Sun.

The Operation of the Pelton Wheel

The mechanical behavior of a spacecraft encountering a moving planet, along its line of motion, is very similar to that of a water jet hitting the edge of a hemispherical cup-vane in a turbine wheel, where its motion is turned around by 180° (drawing).

If the vane does not move, the only effect is to reverse the jet´s direction. Apart from some energy lost to friction, the energy of the jet, and the magnitude of its velocity, stay the same as before.

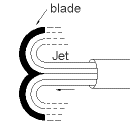Action of the  Pelton wheel

In particular, if the water overtakes a vane moving at half its speed, then (neglecting friction) it loses all its velocity and just dribbles out of the moving vane.

Now total energy is always conserved. Neglecting friction losses (which are converted to heat), the kinetic energy lost by the jet is converted to additional rotational energy of the turbine wheel, speeding up its motion, or perhaps helping run machinery connected to it. By adjusting the speed of the jet to be equal to twice the speed of the vanes, almost all of the jet´s energy can be converted into useful output.

[A problem with the De-Laval turbine is that cannot easily attain this condition. Though the steam jet converts heat energy very efficiently, to efficiently extract energy from the jet, the turbine wheel should match half the jet's velocity. But the jet is supersonic: if it moves at (say) twice the speed of sound, the periphery of the wheel should be moving at the speed of sound, which is rather fast for a power turbine.

 Modern steam turbines use several sets of blades. The first set extracts only part of the energy, and the jet coming out of it is still quite fast. Since the jet now moves in the opposite direction, it must be reversed before it can give more energy to the second blade in line. That is done by a set of stationary blades, firmly attached to the housing of the turbine. As in case #2 above, such blades take away none of the energy.]

Through the later 1870s Pelton developed his design, settling in the end on a double cup with a wedge-shaped divider in the middle, splitting the jet--half to the left, half to the right. (You can approximate that design by cupping your hands upwards, then bringing them together, with the fingernails of one hand touching the ones of the other.) In the winters of 1877 and 1878 he tested turbines of different sizes, including a small one for running his landlady´s sewing machine (it worked, but he was not happy with the design). In 1880 he also obtained a patent on his invention.

Pelton then tried to sell his turbines, but met with little success until the spring of 1883, when the Idaho Mining Company of Grass Valley in Yuba County, California, arranged a competition between different designs, before deciding which design it would buy. Pelton´s turbine won by reaching an efficiency of 90.2%, whereas the three competing water wheels only attained 76.5%, 69.6% and 60.5%. After that sales grew at a tremendous rate, and in 1888 Pelton with some partners formed in San Francisco the Pelton Water Wheel Company, which expanded production even more.